Q.
3x + 2^x – x!! =
x = 6
—————————-
Mapel Matematika, Jenjang Sekolah Menengah Pertama
3x + 2^x – x!!
3(6) + 2⁶ – 6!!
(3 × 6) + (2 × 2 × 2 × 2 × 2 × 2) – (6 × 4 × 2)
18 + (4 × 4 × 4) – (24 × 2)
18 + (16 × 4) – 48
18 + 64 – 48
82 – 48
34
Jawab:
34
Penjelasan dengan langkah-langkah:
Diketahui:
- x = 6
•
Ditanya:
- 3x +
– x!!
•
Dijawab:
=
=
=
=
=
=
=
=
Pertanyaan Baru di Matematika
Ka tolong bantu jawab pertanyaan aku. kasih cara ka
Matematika, Sekolah Dasar
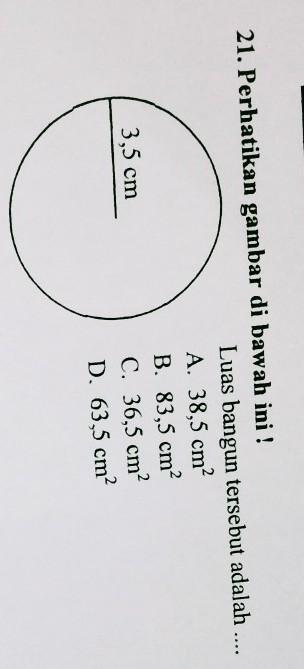
•| LINGKARAN |•
DIKETAHUI :
R : 3,5 CM
DITANYA :
LUAS?
PENYELESAIAN :
L : πr²
L : 22/7 × 3,5 × 3,5
L : 38,5 cm² » a
Diketahui : jari-jari lingkaran = 3,5cm
Ditanya : luas lingkaran ?
Jawab :
L = π × r²
L = 22/7 × 3,5 × 3,5
L = 38,5 cm²
Luas lingkaran dengan jari-jari 3,5 cm adalah 38,5 cm²
Soal :
7! × 17
41 + 89 – 60
5!
otw hapus.
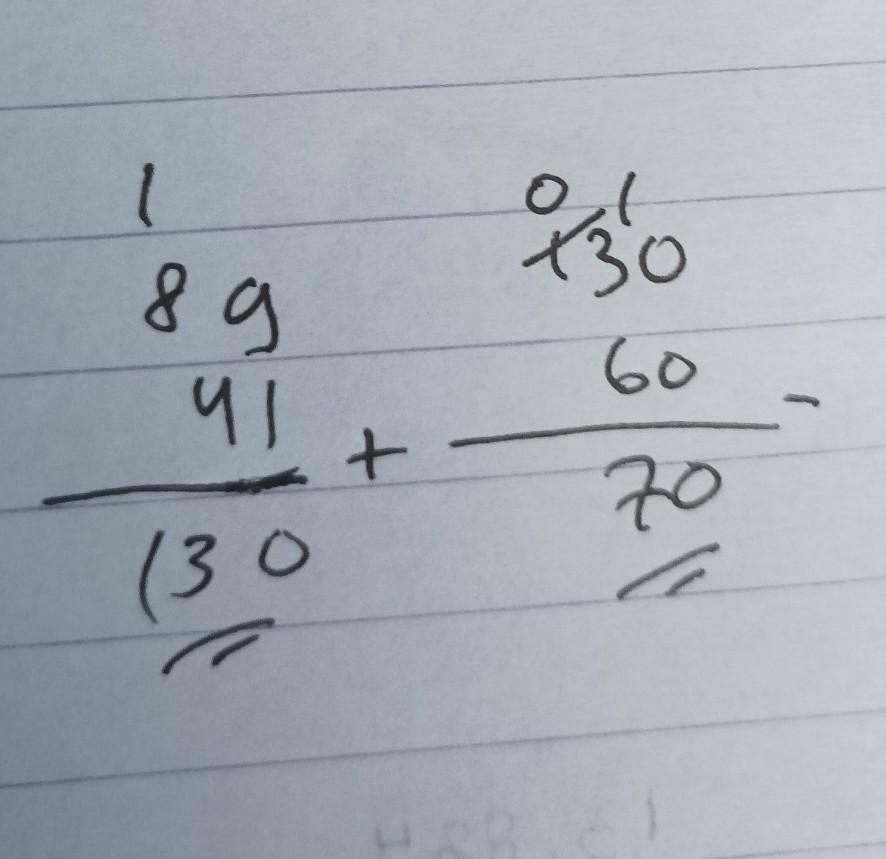
Matematika, Sekolah Menengah Pertama
Soal :
1) 7! × 17 = ?
2) 41 + 89 – 60 = ?
3) 5! = ?
Jawab :
No 1 :
• 7! × 17
• = ( 7 × 6 × 5 × 4 × 3 × 2 × 1 ) × 17
• = ( 42 × 5 × 4 × 3 × 2 × 1 ) × 17
• = ( 42 × 20 × 3 × 2 × 1 ) × 17
• = ( 42 × 20 × 6 × 1 ) × 17
• = ( 840 × 6 × 1 ) × 17
• = ( 5.040 × 1 ) × 17
• = 5.040 × 17
• = 85.680
No 2 :
• 41 + 89 – 60
• = ( 41 + 89 ) – 60
• = 130 – 60
• = 70
No 3 :
• 5!
• = 5 × 4 × 3 × 2 × 1
• = 20 × 3 × 2 × 1
• = 20 × 6 × 1
• = 120 × 1
• = 120
__________________________
Penjelasan dengan langkah-langkah:
= 7! × 17
= (7.6.5.4.3.2.1) × 17
= (42.20.6) × 17
= (42.120) × 17
= 5040 × 17
= 85.680
__
= 41 + 89 – 60
= 130 – 60
= 70
__
= 5!
= 5.4.3.2.1
= 20.6
= 120
Tentukan konstanta dari bentuk aljabar 5×2² – 3x + 7
jawab segera kak !!!
Matematika, Sekolah Menengah Pertama
Jawaban:
5×2² – 3x + 7 =18
Penjelasan dengan langkah-langkah:
5×2² – 3x + 7 =
=5 × 2 × 2 – 3× + 7
=5 × 2 × 2 – 3 – 3 – 3 + 7
=18
SEMOGA MEMBANTU
KALO BOLEH KASIH MAHKOTA YAH MAKASIH
5. Bu Dewi membeli beras 15kg seharga Rp. 150.000, kemudian beras tersebut dijual dengan harga Rp. 12.000/kg. Apabila beras yang terjual sebanyak 8kg. Tentukan : a. Berapakah keuntungan yang di dapatkan bu Dewi?
b. Berapa presentase keuntungan yang diperoleh?
Matematika, Sekolah Dasar
Jawaban:
a.16.000
b.20%
Penjelasan dengan langkah-langkah:
harga beli beras
= 150.000 : 15
= 10.000/kg
harga jual
= 12.000/kg
jumlah keuntungan
=( 12.000 x 8)-(10.000 x 8)
= 96.000 – 80.000
= 16.000
persentase keuntungan
= 16.000/80.000 x 100%
= 20%
3. Dikelas yang terdiri atas 30 siswa, 7 siswa Sudah pernah pergi ke bandung, Il siswa sudah pernah Pergi ke Cirebon A siswa sudah pergi ke bandung dan cirebon banyaknya siswa yang belum ke bandung dan cirebon adalah….! TOLONG DI JAWAB KAK JANGAN ASAL ASALAN KA TERIMA KASIH
Matematika, Sekolah Menengah Atas
✨ Penyelesaian Soal ✨
☛ Soal
Dikelas yang terdiri atas 30 siswa, 7 siswa Sudah pernah pergi ke bandung, II siswa sudah pernah Pergi ke Cirebon A siswa sudah pergi ke bandung dan cirebon banyaknya siswa yang belum ke bandung dan cirebon adalah…
________________
✡ Pendataan
- Diketahui
Jumlah Seluruh Siswa = 30
7 Siswa sudah pernah ke Bandung
II = 2 Siswa sudah pernah ke Cirebon
- Ditanya
Banyak siswa yang belum ke Cirebon dan Bandung -?
- Dijawab
• Menentukan Banyak Siswa yang sudah pernah Ke Cirebon dan Bandung
Cirebon = 2 siswa
Bandung = 7 Siswa
- = Cirebon + Bandung
- = ( 2 + 7 )
- = 9 siswa ☑
• Menentukan Banyak siswa yang Belum pernah Ke Cirebon dan Bandung
• Banyak siswa = Total seluruh Siswa – Banyak siswa yang pernah ke Cirebon dan Bandung
- Banyak siswa = 30 siswa – 9 siswa
- Banyak siswa = 21 Orang siswa ☑
Kesimpulan ☚
- Maka, Banyak siswa yang belum pernah ke Cirebon dan Bandung adalah 21 Orang siswa ☑
☛ Detail Jawaban
- Kelas : 6/SD
- Mapel : Matematika
- Materi : Pendataan dan Statistika
- Kode soal : 2
- Kode kategorisasi : 6.2.1
- Kata Kunci : Banyak, Siswa, Berkunjung, Cirebon, Bandung, Jumlah, Total
Semoga Bisa Bermanfaat-!
#BrainlyMathematic
Penjelasan dengan langkah-langkah:
Ket.
- Total = 30 Siswa
- 7 siswa sudah ke bandung
- 2 siswa sudah pernah ke bandung
—
- Menentukan Banyaknya Siswa Yg Sudah Ke Bandung
= 7 + 2
= 9 siswa
- Menentukan banyaknya siswa yang belum ke bandung dan cirebon.
= Total Siswa – Banyaknya Siswa Yg Sudah Ke Bandung
= 30 – 9
= 21 Yang Belum Ke Bandung
 Desain Grafis Indonesia Kumpulan Berita dan Informasi dari berbagai sumber yang terpercaya
Desain Grafis Indonesia Kumpulan Berita dan Informasi dari berbagai sumber yang terpercaya


